The absolute value function does not have follow any elementary integration rules. We can however bypass this by considering its graph.
This fun integral challenge comes from TMUA, a test by Cambridge University for students looking to study Computer Science at the university 💻
I recommend you pause the article, grab your pen and paper, and give this a go. When you are ready, keep reading for the solution! ✒️
Solution
The trickiest part about this problem is the absolute value function |x|.
What we will do is to split the integral into two, first integrating from x = -1 to x = 0, then from x = 0 to x = 3.

Since the values are negative in the first integral, |x| = -x.
Equally the values are positive in the second integral, so |x| = x.

We then expand the brackets
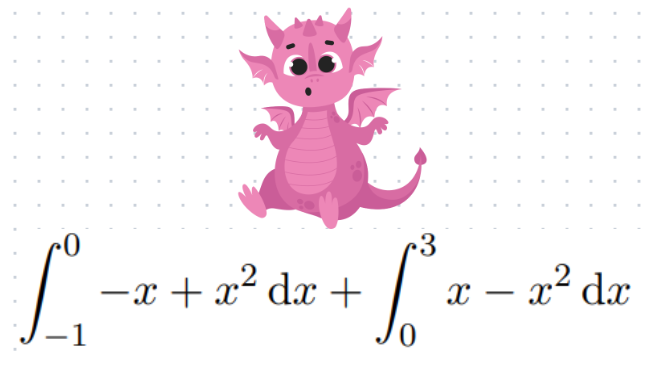
Now I trust that you are capable of integrating using reverse power rule.
And you should get the following!

And that's our answer.
How amazing 🐉
What was your thought process this time? Comment down below, I am eager to know :) 🏹
Save and share the following list for the best math puzzles on Medium👇
Thank you for reading. Don't forget to clap the article if you find it insightful.

I put a lot of time and effort into writing every article for you, so please buy me a coffee☕ if you are feeling generous. It's a great way to support my writing as well as my personal and academic life.
Love, Bella 👑
